Mn dopant on the surface of GaAs

Spin Solotronics

Image of dynamic local exchange interactions in the dc magnetoresistance of spin-polarized current through a dopant

Measurements of the spin excitations of individual atomic spins or small numbers of spins can be performed by measuring the energy threshold for inelastic tunneling processes from a scanning tunneling microscope (STM). These spin excitations depend on the exchange coupling among the spins. For this approach to work the temperature must be sufficiently low that a step increase in the tunneling current can be identified. Still other approaches to measuring interatomic spin interactions require microwave fields that place the spins into a resonant condition, permitting STM-mediated electron spin resonance. Under Department of Energy support, and in collaboration with Ohio State University, we have predicted new fingerprints of the DC spin-polarized current in an STM, in the complete absence of any microwave fields.

In arXiv:1907.05509 we predict strong, dynamical effects in the dc magnetoresistance of current flowing from a spin-polarized electrical contact through a magnetic dopant in a nonmagnetic host. Using the stochastic Liouville formalism we calculate clearly-defined resonances in the dc magnetoresistance when the applied magnetic field matches the exchange interaction with a nearby spin. At these resonances spin precession in the applied magnetic field is canceled by spin evolution in the exchange field, preserving a dynamic bottleneck for spin transport through the dopant. Similar features emerge when the dopant spin is coupled to nearby nuclei through the hyperfine interaction. These features provide a precise means of measuring exchange or hyperfine couplings between localized spins near a surface using spin-polarized scanning tunneling microscopy, without any ac electric or magnetic fields, even when the exchange or hyperfine energy is orders of magnitude smaller than the thermal energy.

This material is based on work supported by the U. S. Department of Energy, Office of Science, Office of Basic Energy Sciences, under Award Number DE-SC0016447.

Figure 1

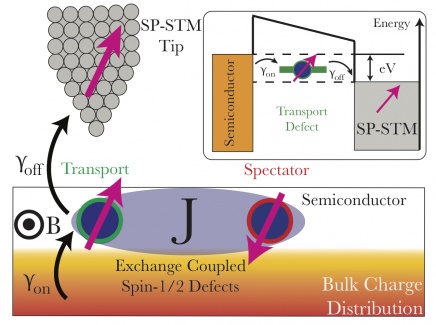
Schematic current path for an electron through a sin- gle dopant (transport site, in green) that is exchange-coupled to another spin-1/2 (spectator site, in red). The transport site has two charge states, empty (spin-0) and full (spin-1/2), and the spectator site’s charge state is stable. These spins reside near the surface of the nonmagnetic host and the Coulomb repulsion at the transport site is assumed to be sufficiently large to prevent double occupation (see inset). Hopping from the transport site to a spin polarized scanning tunneling micro- scope (SP-STM) tip occurs with rate γoff, which is controllable by moving the STM tip relative to the surface. Replenishment of the occupation of the transport site from the nonmagnetic host occurs with a rate γon. If the host is a semiconductor the charge distribution can be adjusted by the STM voltage V through tip induced band bending, which adjusts γon and γoff .

Figure 3

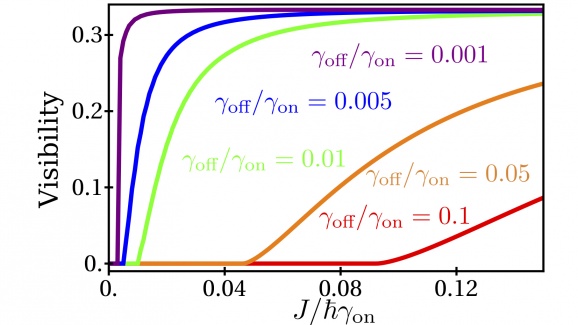
Visibility of the finite-field feature (resonance) as a function of the exchange parameter (J) plotted for different values of the hopping ratio. Smaller hopping ratios yield higher visibility due to extended spin evolution in the exchange field.

Figure 2

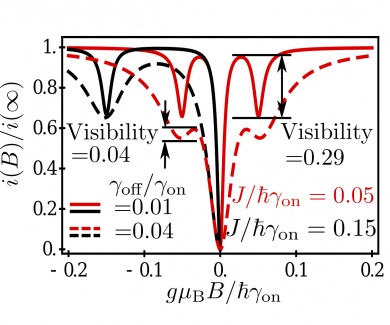
Magnetoresistance of current through spin-1/2 transport dopant for exchange splitting of 0.05 􏰑γon (red) and 0.15 􏰑γon (black). Features broaden for moderate extraction rates (dashed) compared to slow extraction (solid).

Figure4

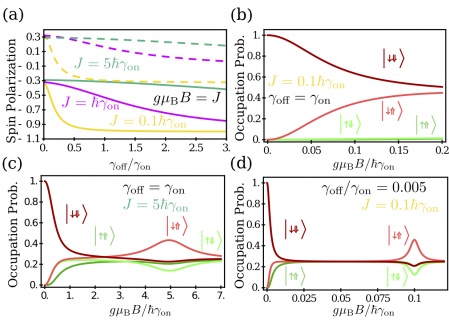
(a) Spin polarization as a function of hopping ra- tio plotted for different values of the exchange coupling. The dashed lines represent the spectator spin and solid lines represent the transport spin. (b-d) Occupation probability for the different states in the product basis as a function of the transverse magnetic field. Shown are (b) γoff = γon, γoff ≫ J, (c)γoff =γon,γoff ≪J,and(d)γon ≫J≫γoff. Good visibility is realized when γoff ≪ J, and especially for (d).